Square and Circle
This page ponders the square and the circle, two shapes, distinct yet intertwined, whispering secrets
through their numbers. I bring no lofty claims, just a quiet fascination and a love for the patterns that
ripple through creation.
Shapes of Harmony
The square stands firm—four equal sides, four right angles, a symbol of stability. Its perimeter is 4s (where
s is the side length), its area s². The circle flows endlessly—one curve, no corners, a breath of eternity. Its
circumference is πd (where d is the diameter), its area πr² (r = d/2). One is finite and grounded; the other,
infinite and free. Yet they meet in a timeless question: can they share the same measure?
Squaring the Circle
To square the circle is to draw a square with the same area as a given circle, using only compass and straight-
edge. Take a circle of radius r: its area is πr². A square of equal area has side length s, where s² = πr²,
so s = r√π. But √π is irrational—unconstructible with finite tools—making the task impossible in classical
terms. Still, the pursuit unveils a bridge: π, the circle’s soul, binds it to the square’s frame.
A Numeric Dance
Set the circle’s circumference equal to the square’s perimeter: πd = 4s. Then s = πd/4, and d = 4s/π.
The diameters align—circle’s d to square’s s—in a ratio of 4/π ≈ 1.273, eerily close to √ɸ (1.272), the golden
ratio’s root. Square that circle’s area (πr², where r = d/2 = 2s/π), and s² ≈ 0.811r²π—a near-echo of unity.
The numbers flirt with harmony, as if teasing a hidden order.
Echoes of Creation
Scripture nods to such shapes: “The city lies foursquare” (Revelation 21:16) meets “He has inscribed a
circle on the face of the deep” (Proverbs 8:27). Square and circle, finite and infinite, reflect a divine duet.
Could their ratios—4/π, √π—be threads in that design, stitching earth to heaven?
This isn’t an answer—it’s a wonder, a spark. I share it for you to ponder, test, or reweave. Numbers are
lanterns—lighting the cosmos, connecting us to its Maker. May this kindle your curiosity and guide you
through the shapes of mystery.
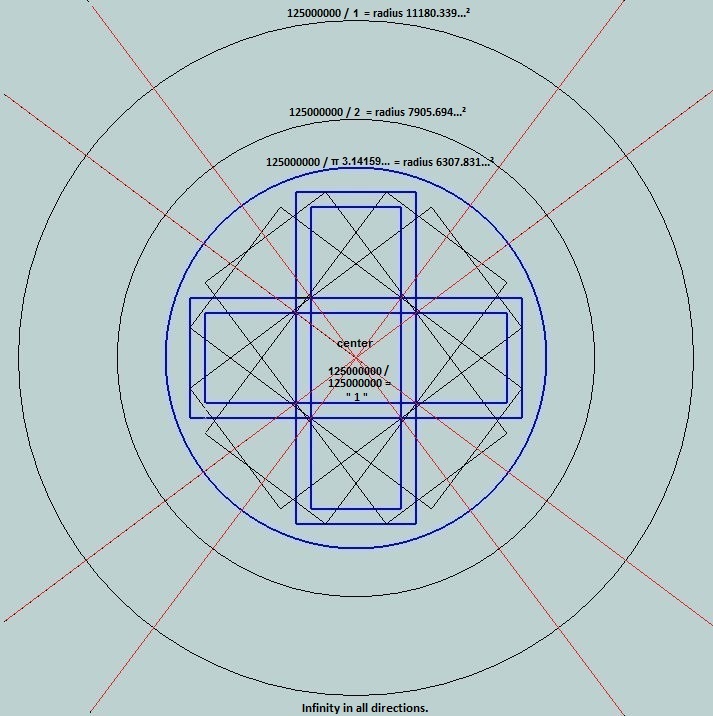
How to make the areas of a square and circle approximately equal to pi, by using Pythagorean Squares
(a continuation from the previous page):
The three circles represent a spiral.
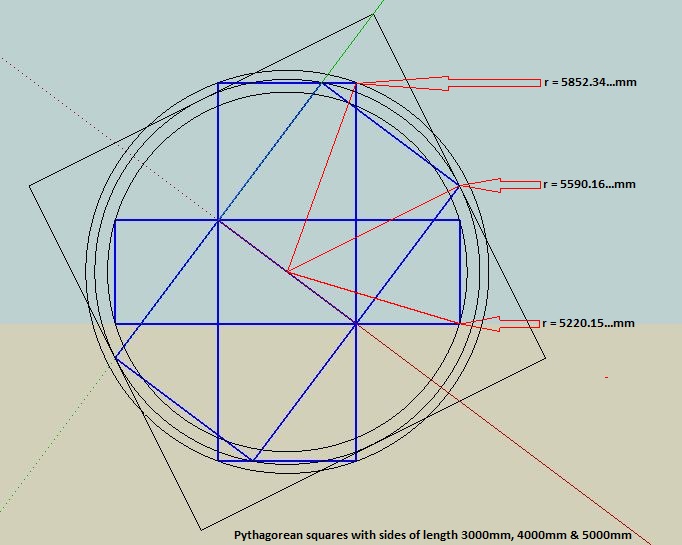
Method 1: Determining the circle radius by using 3 & 7
5852.34...mm2 = 34250000
5590.16...mm2 = 31250000
5220.15...mm2 = 27250000
+ =..................92750000
92750000 / 7 = 13250000
13250000 x 3 = 39750000 (√) = Circle radius 6304.76...mm
Square sides of length 11180.33...mm2 (125000000)
Result: 125000000 / 39750000 = 3.144654088050... (3144654088050 repeats) [4 / 1.272]
4 / 3.144654088050314465408805... = 1.272
4 / 3.144605511029693144278234... = 1.27201964951406896425242...2 = ϕ 1.618033988749... (√1.25 + .5)
4 / 3.141592653589793238462643... = 1.27323954473516268615107... (4 / π)
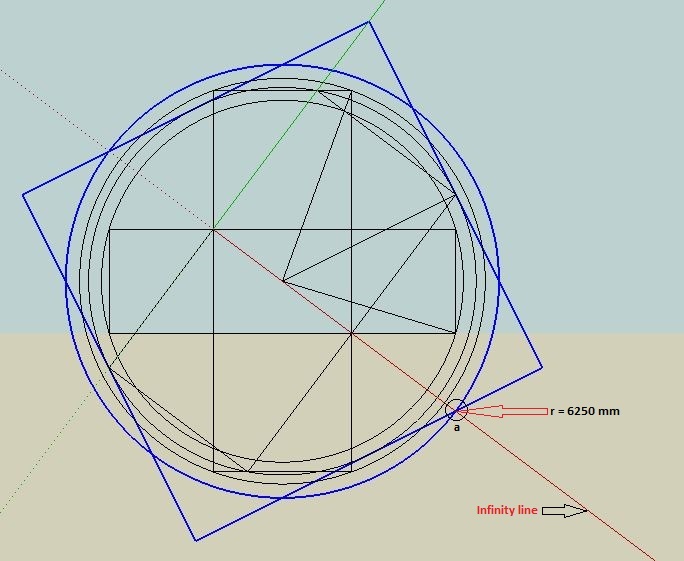
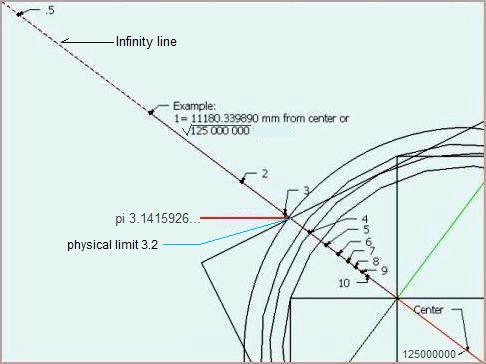
Method 2: Junction between square sides of length and red infinity line = circle radius 6250 mm.
Square sides of length 11180.33...mm 2 (125000000)
Result: 125000000 / 39062500 (6250 mm 2) = 3.2
Circled area " a " enlarged.
Physical limit 3.2
Circled area " b " enlarged.
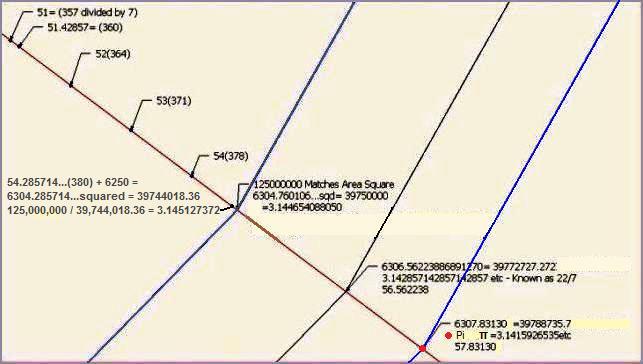
I recalled Noah's Ark dimensions were 300 x 50 x 30= 380, so by following the previous method:
380 / 7 = 54.285714... + 6250 = 6304.285714...2 = 39744018.36
Then 125000000 / 39744018.36 = 3.145127372
This number is close to the blue π line, and it provided the idea for time lines.
Square and Circle Spiral (If r= 1 then L= √ π)
A Square side of (L)ength 11180.3398874989...2 = 125000000
Equivalent:
Circle (r)adius= 6307.8313050504...
Squared =
39788735.7729738339...
Multiplied by
π 3.1415926535...
=
125000000
The orange triangle represents the 5000 mm by 3125 mm Pyramid covered in the Sun Magic Square,
Pi is by Design and Planet diameter pages, and the blue circle represents π 3.1415926535...
From the center working towards π and using seemingly straight linear measurements to the top,
or the four corners converging point of the pyramid at "3.2" signifying the visible physical limit,
we enter the unseen realm. Section 3.2 to π 3.141... shows the illusion of straight lines. All are
arched.
A 3° difference between this Axial tilt method drawing @ 26.565° and Earth's present-day tilt @ 23.5°
Ideas on increasing mass where distance contracts and time is slowed:
The measured distance along the red infinity π line from the center is gradual and even, but when
the radius is squared and divided with the square’s area number 125000000 to get the numbers on
the red infinity line, the numbers increase or slow rapidly depending on the direction of travel.
The spaces between the numbers decrease very fast the closer to the center they get, while
the numbers increase in size.
This acceleration of numbers towards the center, or core, represents the increasing force of
gravity and simultaneous slowing of time, occurring in all forms of substance, ranging from atoms
to planets to galaxies. Whereas increasing number spacings away from mass, represents an
increasing speed of gravity, which may one day be used as a faster way to communicate and
travel. Gravity propagates much faster than the speed of light.
See the increasing and predictable intervals in prime numbers.
So, perhaps the center of mass, an area of no time, is a container for concentrated mass, i.e. light,
i.e. information. And, π may identify c at 186282.397...mps, where light is condensed enough to be
called matter, and show that acceleration of mass creates gravity forces, which creates light waves.
Eight Pythagorean 3-4-5 cubical units uniquely arranged, and a pattern for a beautiful diamond cut.
Three of these (eight 3-4-5 cubical units) form a Sphere. One facing as shown, one oriented
horizontally, and one vertical. It is too difficult for me to draw this, but perhaps you can visualize it.
Fashioned as a Sphere this model reveals an interesting geometric phenomenon.
Plato insisted that squaring the circle (a square equal in area to that of a given circle) can be solved
with a straightedge and compass only.
Continue with squaring the circle.